Fortran - 重回帰式計算（説明変数2個）！

Fortran 95 で、説明（独立）変数2個、目的（従属）変数1個の「重回帰式」を計算する方法についての記録です。

0. 前提条件

• LMDE 3 (Linux Mint Debian Edition 3; 64bit) での作業を想定。
• GCC 6.3.0 (GFortran 6.3.0) でのコンパイルを想定。

1. アルゴリズムについて

当ブログ過去記事を参照のこと。

• Ruby - Array クラス拡張で重回帰式計算！
• Python - 2 つの list から重回帰式計算！
• Python - 2 つの list から重回帰式計算（NumPy 版）！

2. ソースコードの作成

• 連立方程式の解法にはガウスの消去法を使用。

File: regression_multi.f95

!****************************************************
! 重回帰式計算（2元限定）
!
!   date          name            version
!   2018.12.18    mk-mode.com     1.00 新規作成
!
! Copyright(C) 2018 mk-mode.com All Rights Reserved.
!****************************************************
!
module const
  ! SP: 単精度(4), DP: 倍精度(8)
  integer,     parameter :: SP = kind(1.0)
  integer(SP), parameter :: DP = selected_real_kind(2 * precision(1.0_SP))
end module const

module comp
  use const
  implicit none
  private
  public :: calc_reg_multi

contains
  ! 重回帰式計算
  !
  ! :param(in)  real(8) x(:, 2): 説明変数配列
  ! :param(in)  real(8)    y(:): 目的変数配列
  ! :param(out) real(8)       c: 定数
  ! :param(out) real(8)    v(2): 係数
  subroutine calc_reg_multi(x, y, c, v)
    implicit none
    real(DP), intent(in)  :: x(:, :), y(:)
    real(DP), intent(out) :: c, v(2)
    integer(SP) :: size_x, size_y, i
    real(DP)    :: mtx(3, 3), mtx_2(2, 3)

    size_x = size(x) / 2
    size_y = size(y)
    if (size_x == 0 .or. size_y == 0) then
      print *, "[ERROR] array size == 0"
      stop
    end if
    if (size_x /= size_y) then
      print *, "[ERROR] size(X) != size(Y)"
      stop
    end if

    mtx(1, 1) = sum_p(y, y)
    mtx(2, 1) = sum_p(y, x(:, 1))
    mtx(3, 1) = sum_p(y, x(:, 2))
    do i = 1, 2
      mtx(1, i + 1) = sum_p(x(:, i), y)
      mtx(2, i + 1) = sum_p(x(:, i), x(:, 1))
      mtx(3, i + 1) = sum_p(x(:, i), x(:, 2))
    end do
    mtx_2 = transpose(reshape((/ &
      & mtx(2, 2), mtx(3, 2), mtx(1, 2), &
      & mtx(2, 3), mtx(3, 3), mtx(1, 3)  &
    & /), (/3, 2/)))
    call gauss_e(2, mtx_2)
    v = (/mtx_2(1, 3), mtx_2(2, 3)/)
    c = calc_const(reshape((/y(:), x(:, 1), x(:, 2)/), (/size_x, 3/)), v)
  end subroutine calc_reg_multi

  ! Sum-of-producsts computation
  !
  ! :param(in) real(8)  x(:): 実数配列
  ! :param(in) real(8)  y(:): 実数配列
  ! :retrun    real(8) sum_p: sum of products
  real(DP) function sum_p(x, y)
    implicit none
    real(DP), intent(in) :: x(:), y(:)
    integer(SP) :: size_x, size_y, i
    real(DP)    :: avg_x, avg_y

    size_x = size(x)
    size_y = size(y)
    avg_x = sum(x) / size_x
    avg_y = sum(y) / size_y
    sum_p = sum((/((x(i) - avg_x) * (y(i) - avg_y), i=1,size_x)/))
  end function sum_p

  ! Gaussian elimination
  !
  ! :param(in)    integer(4)     n: 元数
  ! :param(inout) real(8) a(n,n+1): 係数配列
  subroutine gauss_e(n, a)
    implicit none
    integer(SP), intent(in)    :: n
    real(DP),    intent(inout) :: a(n, n + 1)
    integer(SP) :: i, j
    real(DP)    :: d

    ! 前進消去
    do j = 1, n - 1
      do i = j + 1, n
        d = a(i, j) / a(j, j)
        a(i, j+1:n+1) = a(i, j+1:n+1) - a(j, j+1:n+1) * d
      end do
    end do

    ! 後退代入
    do i = n, 1, -1
      d = a(i, n + 1)
      do j = i + 1, n
        d = d - a(i, j) * a(j, n + 1)
      end do
      a(i, n + 1) = d / a(i, i)
    end do
  end subroutine gauss_e

  ! Constant term computation
  !
  ! :param(in) real(8) a(:, 3): 配列（目的変数, 説明変数1, 説明変数2）
  ! :param(in) real(8)    v(2): 係数
  ! :return    real(8)       c: 定数項
  function calc_const(a, v) result(c)
    implicit none
    real(DP), intent(in) :: a(:, :), v(:)
    real(DP) :: c
    integer(SP) :: size_a, i
    real(DP)    :: s(3)

    size_a = size(a(:,1))
    s = (/(sum(a(:, i)), i=1,3)/)
    c = s(1) / size_a
    do i = 2, size_a
      c = c - s(i) * v(i - 1) / size_a
    end do
  end function calc_const
end module comp

program regression_multi
  use const
  use comp
  implicit none
  real(DP)    :: x(10, 2), y(10), c, v(2)
  integer(SP) :: i

  x = reshape((/ &
    & 17.5_DP, 17.0_DP, 18.5_DP, 16.0_DP, 19.0_DP, &
    & 19.5_DP, 16.0_DP, 18.0_DP, 19.0_DP, 19.5_DP, &
    & 30.0_DP, 25.0_DP, 20.0_DP, 30.0_DP, 45.0_DP, &
    & 35.0_DP, 25.0_DP, 35.0_DP, 35.0_DP, 40.0_DP  &
  & /), (/10, 2/))
  y = (/ &
    & 45.0_DP, 38.0_DP, 41.0_DP, 34.0_DP, 59.0_DP, &
    & 47.0_DP, 35.0_DP, 43.0_DP, 54.0_DP, 52.0_DP  &
  & /)

  do i = 1, 2
    print '(A, I0, A, 10F8.2, A)', "説明変数 X(", i, ") = (", x(:, i), ")"
  end do
  print '(A, 10F8.2, A)', "目的変数 Y    = (", y, ")"
  print '(A)', "---"

  call calc_reg_multi(x, y, c, v)
  print '(A, F14.8)', "定数項 = ", c
  print '(A, F14.8)', "係数-1 = ", v(1)
  print '(A, F14.8)', "係数-2 = ", v(2)
end program regression_multi

• Gist - Fortran 95 source code to compute multiple regression equations.

3. ソースコードのコンパイル

$ gfortran -o regression_multi regression_multi.f95

4. 動作確認

$ ./regression_multi
説明変数 X(1) = (   17.50   17.00   18.50   16.00   19.00   19.50   16.00   18.00   19.00   19.50)
説明変数 X(2) = (   30.00   25.00   20.00   30.00   45.00   35.00   25.00   35.00   35.00   40.00)
目的変数 Y    = (   45.00   38.00   41.00   34.00   59.00   47.00   35.00   43.00   54.00   52.00)
---
定数項 =   -34.71293084
係数-1 =     3.46981263
係数-2 =     0.53300948

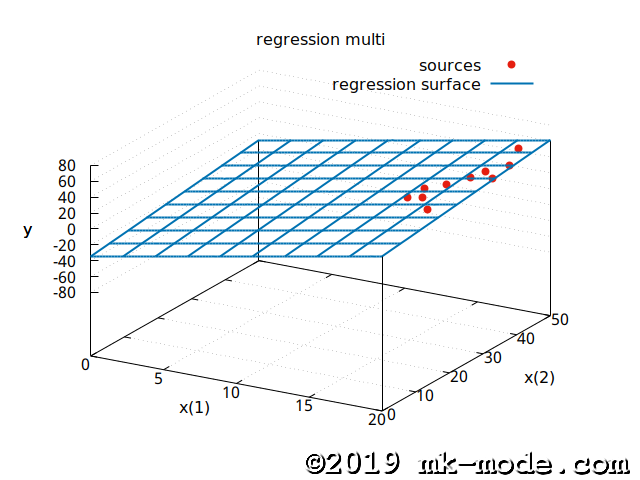
求まった回帰曲面は

\[y = -34.71293084 + 3.46981263 x_1 + 0.53300948 x_2\]

ということである。

参考までに、回帰曲面と点を gnuplot で描画してみた。（説明変数 \(x_1, x_2\) を \(x, y\) 軸、目的変数 \(y\) を \(z\) 軸としてしている）（若干、分かりにくいかも）

---

以上。